An alternative means of determining the area of a trapezoid involves breaking the trapezoid into a triangle and a rectangle. The areas of the triangle and rectangle can be computed individually; the area of the trapezoid is then the sum of the areas of the triangle and the rectangle. This method is illustrated in the graphic below.

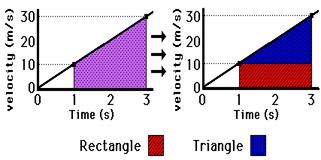
Triangle: Area = ½ * (2 s) * (20 m/s) = 20 m

Rectangle: Area = (2 s) * (10 m/s) = 20 m

Total Area = 20 m + 20 m = 40 m

It has been learned in this lesson that the area bounded by the line and the axes of a velocity-time graph is equal to the displacement of an object during that particular time period. The area can be identified as a rectangle, triangle, or trapezoid. The area can be subsequently determined using the appropriate formula. Once calculated, this area represents the displacement of the object.