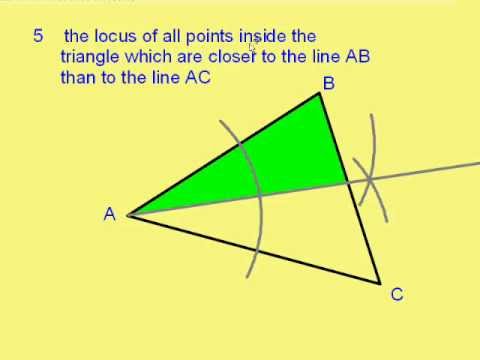
Problem 2.1 Loci of Points – OBA is a simple crank chain. OB is a crank of 35 mm length. BA is connecting rod of 90 mm length. Slider A is sliding on a straight path passing through the point O. Draw the locus of the mid-point of the connecting rod AB for one complete revolution of the crank OB.
Procedure:
Step-1 First draw a horizontal center line of sufficient length.
Step-2 Draw a vertical center line and mark point O at the intersection of the two center lines.
Step-3 Draw a circle by taking point O as center of radius equal to the length of crack OB, which is 35 mm.
Step-4 Divide the circle into 12 or 8 equal divisions. And mark the points B1, B2, B3 etc. up to B12 as given into the figure. Note: Numbering should be given in the direction of rotation of crank OB. (Clockwise or Anticlockwise)
Step-5 Draw straight lines of length equal to the length of connecting rod AB, which is 90 mm long, from the point B1, B2, B3, etc. such that these lines should connect on the horizontal center line. And give the numbers A1, A2, A3 etc. in sequence as depicted into the figure.
Step-6 Mark center points of lines A1B1, A2B2, A3B3 etc. and give the numbers 1,2,3,4, etc. shown into the figure.
Step-7 Now connects the points 1,2,3, etc. in sequence by a free hand medium dark curve.
Step-8 Give the dimensions and name of components as shown into the figure.
Step-9 Give the dimensions by any one method of dimensions and give the name of the components by leader lines wherever necessary.