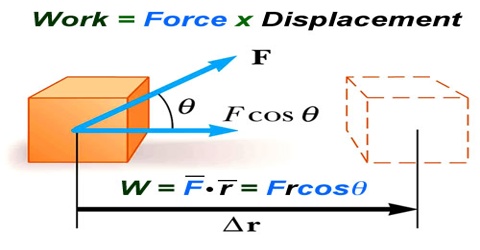
In a previous topic, work was described as taking place when a force acts upon an object to cause a displacement. When a force acts to cause an object to be displaced, three quantities must be known in order to calculate the work. Those three quantities are force, displacement and the angle between the force and the displacement. The work is subsequently calculated as force•displacement•cosine (theta) where theta is the angle between the force and the displacement vectors. In this part of Lesson 1, the concepts and mathematics of work will be applied in order to analyze a variety of physical situations.
Check Your Understanding
Express your understanding of the concept and mathematics of work by answering the following questions. When done, click the button to view the answers.
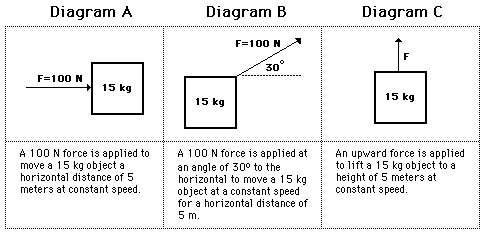
1. Apply the work equation to determine the amount of work done by the applied force in each of the three situations described below.
See Answer

Diagram A Answer:
W = (100 N) * (5 m)* cos(0 degrees) = 500 J
The force and the displacement are given in the problem statement. It is said (or shown or implied) that the force and the displacement are both rightward. Since F and d are in the same direction, the angle is 0 degrees.
Diagram B Answer:
W = (100 N) * (5 m) * cos(30 degrees) = 433 J
The force and the displacement are given in the problem statement. It is said that the displacement is rightward. It is shown that the force is 30 degrees above the horizontal. Thus, the angle between F and d is 30 degrees.
Diagram C Answer:
W = (147 N) * (5 m) * cos(0 degrees) = 735 J
The displacement is given in the problem statement. The applied force must be 147 N since the 15-kg mass (Fgrav=147 N) is lifted at constant speed. Since F and d are in the same direction, the angle is 0 degrees.
2. On many occasions, there is more than one force acting upon an object. A free-body diagram is a diagram that depicts the type and the direction of all the forces acting upon an object. The following descriptions and their accompanying free-body diagrams show the forces acting upon an object. For each case, indicate which force(s) are doing work upon the object. Then calculate the work done by these forces.
| Free-BodyDiagram | Forces Doing Workon the Object | Amount of Work Doneby Each Force |
A 10-N force is applied to push a block across a friction free surface for a displacement of 5.0 m to the right. | See Answer | See Answer |
A 10-N frictional force slows a moving block to a stop after a displacement of 5.0 m to the right.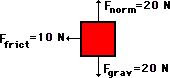 | See Answer | See Answer |
A 10-N force is applied to push a block across a frictional surface at constant speed for a displacement of 5.0 m to the right. | See Answer | See Answer |
An approximately 2-kg object is sliding at constant speed across a friction free surface for a displacement of 5 m to the right.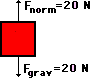 | See Answer | See Answer |
An approximately 2-kg object is pulled upward at constant speed by a 20-N force for a vertical displacement of 5 m. | See Answer | See Answer |
3. Before beginning its initial descent, a roller coaster car is always pulled up the first hill to a high initial height. Work is done on the car (usually by a chain) to achieve this initial height. A coaster designer is considering three different incline angles at which to drag the 2000-kg car train to the top of the 60-meter high hill. In each case, the force applied to the car will be applied parallel to the hill. Her critical question is:which angle would require the most work? Analyze the data, determine the work done in each case, and answer this critical question.
| Angle | Force | Distance | Work (J) | |
| a. | 35 deg | 1.12 x 104 N | 105 m | |
| b. | 45 deg | 1.39 x 104 N | 84.9 m | |
| c. | 55 deg | 1.61 x 104 N | 73.2 m |
See Answer

Be careful!
The angle in the table is the incline angle. The angle theta in the equation is the angle between F and d. If the F is parallel to the incline and the d is parallel to the incline, then the angle theta in the work equation is 0 degrees. For this reason, W=F*d*cosine 0 degrees.
In each case, the work is approximately 1.18 x106 Joules.
The angle does not affect the amount of work done on the roller coaster car.
4. Ben Travlun carries a 200-N suitcase up three flights of stairs (a height of 10.0 m) and then pushes it with a horizontal force of 50.0 N at a constant speed of 0.5 m/s for a horizontal distance of 35.0 meters. How much work does Ben do on his suitcase during this entire motion?
See Answer

The motion has two parts: pulling vertically to displace the suitcase vertically (angle = 0 degrees) and pushing horizontally to displace the suitcase horizontally (angle = 0 degrees).
For the vertical part, W = (200 N) * (10 m) * cos (0 deg) = 2000 J.
For the horizontal part, W = (50 N) * (35 m) * cos (0 deg) = 1750 J.
The total work done is 3750 J (the sum of the two parts).
close
5. A force of 50 N acts on the block at the angle shown in the diagram. The block moves a horizontal distance of 3.0 m. How much work is done by the applied force?
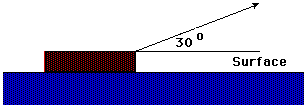
See Answer
W = F * d * cos(Theta)
W = (50 N) * (3 m) * cos (30 degrees) = 129.9 Joules
6. How much work is done by an applied force to lift a 15-Newton block 3.0 meters vertically at a constant speed?
See Answer

To lift a 15-Newton block at constant speed, 15-N of force must be applied to it (Newton’s laws). Thus,
W = (15 N) * (3 m) * cos (0 degrees) = 45 Joules
7. A student with a mass of 80.0 kg runs up three flights of stairs in 12.0 sec. The student has gone a vertical distance of 8.0 m. Determine the amount of work done by the student to elevate his body to this height. Assume that his speed is constant.
See Answer

The student weighs 784 N (Fgrav= 80 kg * 9.8 m/s/s).
To lift a 784-Newton person at constant speed, 784 N of force must be applied to it (Newton’s laws). The force is up, the displacement is up, and so the angle theta in the work equation is 0 degrees. Thus,
W = (784 N) * (8 m) * cos (0 degrees) = 6272 Joules
| 8. Calculate the work done by a 2.0-N force (directed at a 30° angle to the vertical) to move a 500 gram box a horizontal distance of 400 cm across a rough floor at a constant speed of 0.5 m/s. (HINT: Be cautious with the units.) |  |
See Answer

Here is a good example of the importance of understanding the angle between F and d. In this problem, the d is horizontal and the F is at a 60-degree angle to the horizontal. Thus, theta is 60 degrees.
W = (2.0 N) * (4.00 m) * cos (60 degrees) = 4.0 J
9. A tired squirrel (mass of 1 kg) does push-ups by applying a force to elevate its center-of-mass by 5 cm. Estimate the number of push-ups that a tired squirrel must do in order to do a approximately 5.0 Joules of work.
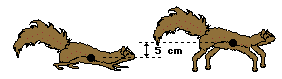
See Answer

The squirrel applies a force of approximately 10 N (9.8 N to be exact) to raise its body at constant speed. The displacement is 0.05 meters. The angle between the upward force and the upward displacement is 0 degrees. The work for 1 push-up is approximately
W = 10 N * 0.05 m * cos 0 degrees = 0.5 Joules
If the squirrel does a total of 5.0 Joules of work, then it must have done about 10 push-ups.