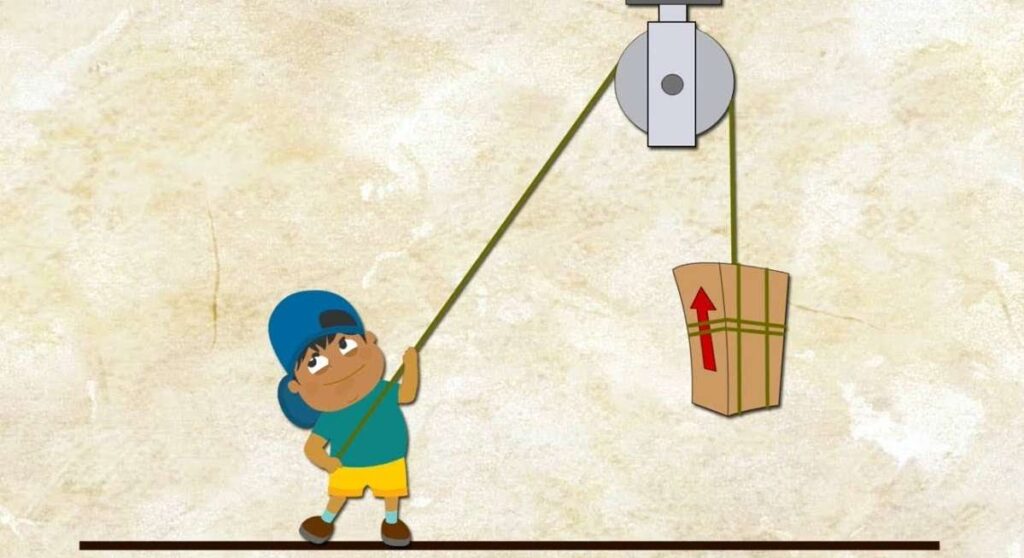
Sometimes the simplest alteration in design results in a huge improvement, a truth I’ve discovered more than a few times during my years as an engineering expert. Last time we introduced the simple pulley and revealed that its usefulness was limited to the strength of the pulling force behind it. Hundreds of years ago that force was most often supplied by a man and his biceps. But ancient Greeks found an ingenious and simple way around this limitation, which we’ll highlight today by way of a modern design engineer’s tool, the free body diagram. Around 400 BC, the Greeks noticed that if they detached the simple pulley from the beam it was affixed to in our last blog and instead allowed it to be suspended in space with one of its rope ends fastened to a beam, the other rope end to a pulling force, something interesting happened.  The Simple Pulley Improved It was much easier to lift objects while suspended in air. As a matter of fact, it took 50% less effort. To understand why, let’s examine what engineers call a free body diagram of the pulley in our application, as shown in the blue inset box and in greater detail below. The Simple Pulley Improved It was much easier to lift objects while suspended in air. As a matter of fact, it took 50% less effort. To understand why, let’s examine what engineers call a free body diagram of the pulley in our application, as shown in the blue inset box and in greater detail below. Using a Free Body Diagram to Understand Simple Pulleys The blue insert box in the first illustration highlights the subject at hand. A free body diagram helps engineers analyze forces acting upon a stationary object suspended in space. The forces acting upon the object, in our case a simple pulley, represent both positive and negative values. The free body diagram above indicates that forces pointing up are, by engineering convention, considered to be positive, while downward forces are negative. The basic rule of all free body diagrams is that in order for an object to remain suspended in a fixed position in space, the sum of all forces acting upon it must equal zero. We’ll see how the free body diagram concept is instrumental in understanding the improvement upon the action of a simple pulley next time, when we attack the math behind it. Using a Free Body Diagram to Understand Simple Pulleys The blue insert box in the first illustration highlights the subject at hand. A free body diagram helps engineers analyze forces acting upon a stationary object suspended in space. The forces acting upon the object, in our case a simple pulley, represent both positive and negative values. The free body diagram above indicates that forces pointing up are, by engineering convention, considered to be positive, while downward forces are negative. The basic rule of all free body diagrams is that in order for an object to remain suspended in a fixed position in space, the sum of all forces acting upon it must equal zero. We’ll see how the free body diagram concept is instrumental in understanding the improvement upon the action of a simple pulley next time, when we attack the math behind it. |
Posted inStrength of Materials