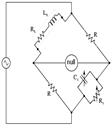
The bridge circuit shown above is known as the Maxwell-Wien bridge (often called the Maxwell bridge), and is used to measure unknown inductances in terms of calibrated resistance and capacitance. Calibration-grade inductors are more difficult to manufacture than capacitors of similar precision, and so the use of a simple “symmetrical” inductance bridge is not always practical. Because the phase shifts of inductors and capacitors are exactly opposite each other, a capacitive impedance can balance out an inductive impedance if they are located in opposite legs of a bridge, as they are here.

Unlike this straight Wien bridge, the balance of the Maxwell-Wien bridge is independent of the source frequency. In some cases, this bridge can be made to balance in the presence of mixed frequencies from the AC voltage source, the limiting factor being the inductor’s stability over a wide frequency range.

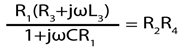
Using the equations above you can calculate appropriate values for C and R2 for a set of probe values. Then, using your calculated values, balance the bridge. The oscilloscope trace representing current (brightest green) across the top and bottom of the bridge should be minimized (straight line).

In the simplest implementation, the standard capacitor (C) and the resistor in parallel with it are made variable, and both must be adjusted to achieve balance. However, the bridge can be made to work if the capacitor is fixed (non-variable) and more than one resistor is made variable (at least the resistor in parallel with the capacitor, and one of the other two). However, in the latter configuration it takes more trial-and-error adjustment to achieve balance as the different variable resistors interact in balancing magnitude and phase.

Another advantage of using a Maxwell bridge to measure inductance rather than a symmetrical inductance bridge is the elimination of measurement error due to the mutual inductance between the two inductors. Magnetic fields can be difficult to shield, and even a small amount of coupling between coils in a bridge can introduce substantial errors in certain conditions. With no second inductor to react within the Maxwell bridge, this problem is eliminated.