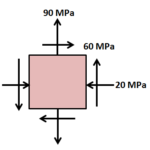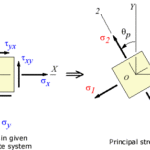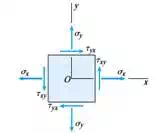
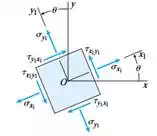
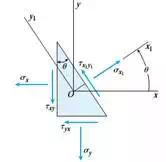
a.       The stresses acting on the inclined x₁y₁ element can be expressed in terms of the stresses on the xy element by using equations of equilibrium

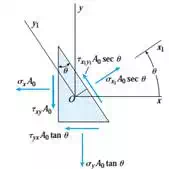
b.      Let us denote the area of the left-hand side face (that is, the negative x face) as A₀

Summing forces in the x₁ direction

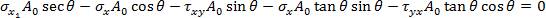
Summing forces in the y₁ direction

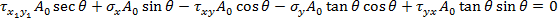
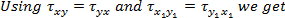
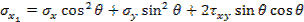
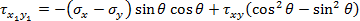
c.    Transformation Equations for Plane Stress

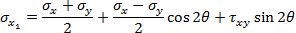
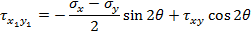
                                                              i.      Transformation equations were derived solely from equilibrium of an element, they are applicable to stresses in any kind of material, whether linear or nonlinear, elastic or inelastic

                                                            ii.      can be obtained by putting  as 

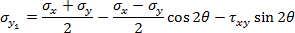
d.      Few Important points

                                                              i.      Only one intrinsic state of stress exists at a point in a stressed body, regardless of the orientation of the element being used to portray that state of stress. When we have two elements with different orientations at the same point in a body, the stresses acting on the faces of the two elements are different, but they still represent the same state of stress, namely, the stress at the point under consideration. This situation is analogous to the representation of a force vector by its components— although the components are different when the coordinate axes are rotated to a new position, the force itself is the same.

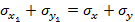
The above equation can be obtained by adding 

                                                            ii.      The shear stresses acting on all four side faces of an element in plane stress are known if we determine the shear stress acting on any one of those faces.