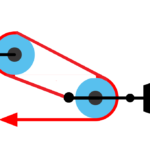
 Dynamic Lifting is Easier With a Compound PulleyIf you’ll recall from our last topic, Mr. Toga used a compound pulley to assist him in holding an urn stationary in space. To do so, he only needed to exert personal bicep force, F, equivalent to half the urn’s weight force, W, which meant he enjoyed a mechanical advantage of 2. Mathematically that is represented by,F = W ÷ 2If the urn weighs 40 pounds, then he only needs to exert 20 Lbs of personal effort to keep it suspended. But when Mr. Toga uses more bicep power with that same compound pulley, he’s able to dynamically raise its position in space until it eventually meets with the beam that supports it. All the while he’ll be exerting a force greater than W ÷ 2. That relationship is represented by,F > W ÷ 2 In the case of a 40 Lb urn, the lifting force Mr. Toga must exert to dynamically lift the urn is represented by,F > 40 Lbs ÷ 2F > 20 Lbswhere F represents a bicep force of at least 20 pounds. Fortunately for him, his efforts will never have to extend much beyond 20 Lbs of effort to lift the urn to the beam. That’s because gravity’s effect will remain nearly constant as the urn climbs, this being due to gravity’s influence upon objects decreasing by an insignificant amount over short distances above the Earth’s surface. As a matter of fact, at an altitude of 3,280 feet, gravity’s pull decreases by a mere 0.2 %. The net result is that the compound pulley enables the same mechanical advantage whether a static or dynamic scenario exists, that is, regardless of whether Mr. Toga is simply holding the urn stationary in space or he’s actively tugging on his end of the rope to lift it higher. |
Comments are closed.