Modulus of elasticity () and the modulus of rigidity () are related by the following equation:

Here,  represents a number called Poisson’s ratio given to the particular material. When the material is being stretched in one direction, it gets shortened in a perpendicular direction. In the direction that the material becomes elongated, the axial strain () is defined as the fractional increase in the length. In the direction that the material shortens, the transverse strain () gives the fractional reduction in length. The diagram below illustrates these changes in shape:

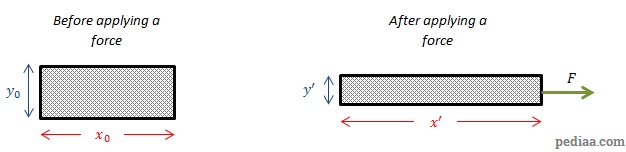
Defining Poisson’s ratio

In this diagram, the axial strain is:

The transverse strain is:

Note that since the object shortens in the direction perpendicular to the force, . Poisson’s ratio () is defined as:

The minus sign has been introduced to ensure that  takes a positive value.