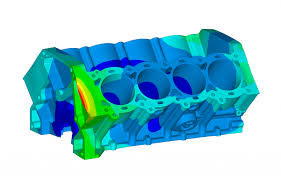
When performing complex finite element analyses, engineers typically bypass making the simplest, yet often most valuable, analytical model that can be created. They often waste a significant amount of time debugging creep or hyperelastic analyses that have material models that could easily be shown to be invalid with single element analytical testing. Making a one element model may sound like a waste of time, but most FEA experts use these on a regular basis.

There are a number of possible uses of the single element model, including:

●     Testing nonlinear material models

●     Testing of macros and user-defined routines

●     Evaluation of the impact of large aspect ratios, skew angles or warped elements

●     A single element model can be defined with a link, beam, 2-d solid, 3-d brick or tetrahedron. Exercising the same element proposed in the full analyses yields the additional validation that the proposed material law and convergence is valid for this element formulation.

When modeling a single brick for material validation, I like to use symmetry boundary conditions on three orthogonal faces, and then a non-zero displacement imposed on one of the free faces. This method creates a constant stress and strain state in the single element. The use of an incremental displacement based loading approach provides a quick and stable solution. The use of a single coupling equation to tie all the imposed displacement degree of freedom nodes together, allows one to postprocess the time history of the total force reaction from the master node.

If one is evaluating a hyperelastic material law where large deformations are active, plotting stress vs. strain provides the true stress vs. log strain results that can be compared to any existing test data.

Other loading checks that can be performed, depending upon the analysis goals, include:

●     The evaluation of temperature-dependent material properties through ramped temperature loading.

●     The evaluation of creep laws with either a load-based simulation to calculate the increased deformation over time, or a displacement based solution to predict stress relaxation.

●     All of these analyses can be repeated with distorted element shapes. This approach can be valuable for predicting stress error and/or evaluating convergence efficiency for irregular elements.